Hardware Modules > aMG SIGCON-A > Signal Conditioning Design Guidline > Sample Applications

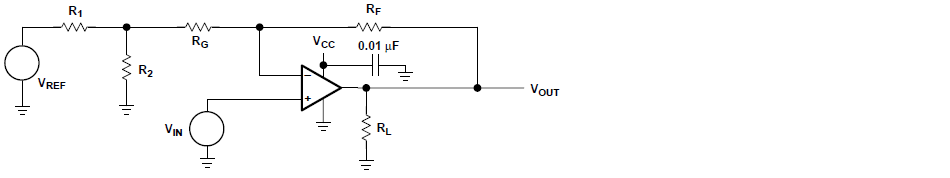
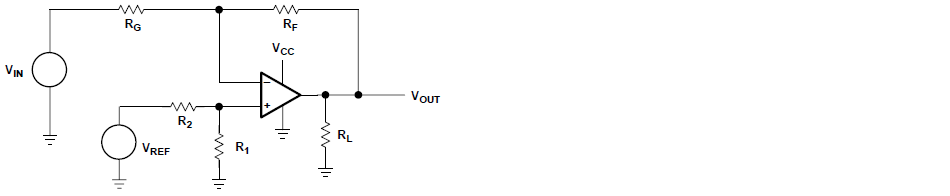
The zero and span circuit is frequently used in instrumentation and measurement processes. This circuit adjust the slope and zero point of the signal which correlation between input and output.

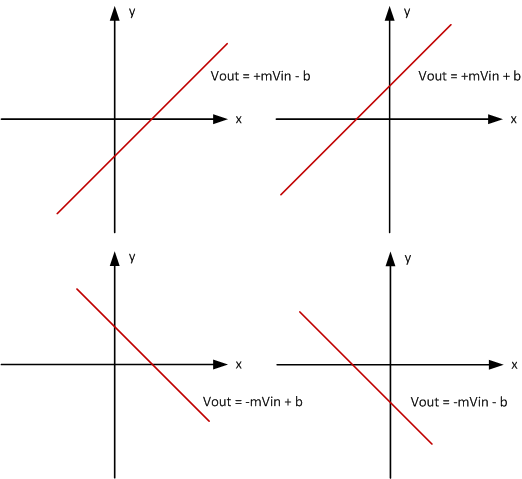
A linear op amp transfer function is limited to the equation of a straight line (Eq1)

y = +-mx +- b (1)

The equation of a straight line has four possible solutions depending upon the sign of m (slope) and b (intercept), thus the equations yield solutions in four forms.

CASE1: Vout = +mVin + b (2)

CASE2: Vout = +mVin - b (3)

CASE3: Vout = -mVin + b (4)

CASE4: Vout = -mVin - b (5)

transferCurve

Four circuits must be developed follow-up to the equation CASE1 to CASE4:

CASE1: Vout = +mVin + b

span_case1

CASE2: Vout = +mVin - b

span_case2

CASE3: Vout = -mVin + b

span_case3

CASE4: Vout = -mVin - b

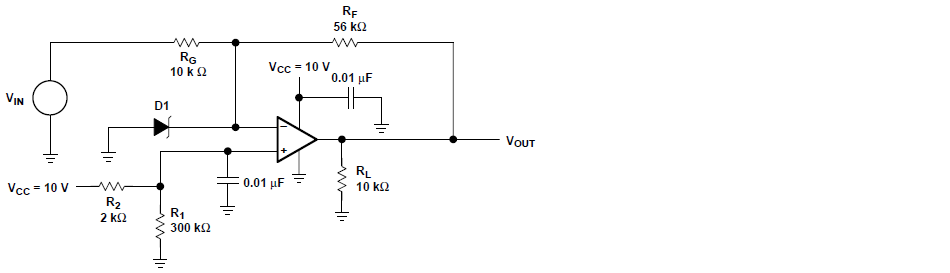
span_case4

Example: Given a set of two data points for Vout and Vin, and equations are solved to determine m and b for the equation that satisfies the given data; i. e., a sensor output signal ranging from 0.1 V to 0.2 V must be interfaced into an analog-to-digital converter that has an input voltage range of 1 V to 4 V.

These data points (Vout = 1 V @ Vin = 0.1 V, Vout = 4 V @ Vin = 0.2 V) are inserted into Equation 2 (CASE 1), to obtain m and b for the specifications.

1 = m(0.1) + b (6)

4 = m(0.2) + b (7)

After algebraic manipulation of Equation 6, 7, yield the solution, m = 30 and b = -2.

Now m and b are rewrite to new equation.

Vout = 30Vin - 2 (8)

The equation (8) similar to the CASE2: Vout = +mVin - b circuit. The next step required to complete the problem solution is to develop a circuit that has an m = 30 and b = –2 (CASE 2).